Extra Credit #1

Begins Monday, Oct 7, 2024

Ends Thursday, Oct 17, 2024

The purpose of this assignment is to allow students to demonstrate their understanding of various abstract and applied physics topics. Because of the nature of this assignment, it will be graded fairly strictly—pay close attention to the Notes below.

NOTES:

1. The Extra Credit Assignment consists of four problems, to be completed outside of class.
2. This assignment is Extra Credit and not required.
3. This assignment is intended to provide students with the opportunity to review some of the material we've covered, and not all students need the same amount of review. Students will be eligible to receive credit on problems completed according to the table below.

   Your Test Average (incl. Ch 5-6 test)	Review Problems You Are Eligible to Complete
   0 - 79%	Problems #1 - 4
   80 - 89%	Problems #2 - 4
   90 - 100%	Problems #3 - 4

4. The total points earned by a student on this assignment will be determined by the instructor based on a number of factors, including number of problems completed, difficulty of problem, and overall quality of assignments submitted by students.
5. You may talk with other students about these problems and how to go about solving them—in fact, this is encouraged—but each person must complete their own assignment to be turned in, without looking at other students' work. Even when numeric answers to problems are the same, solutions from different students must be written independently, and differently, and developed and explained in each student's own words.
6. The assignment must have a separate cover sheet that includes your Name, Date, Course and Period, and Name of Assignment. Staple this cover sheet to the front of the problems that you've solved.
7. Each response must be hand-written on a separate piece of paper, which must include:

   a) Your name and the problem number at the top of the page.
   b) Salient details from the original question written out as appropriate.
   c) Your solution, hand-written in blue ink, black ink, or dark pencil, with all work shown in detail.
   d) Drawings, diagrams, or graphs with labels must be used to explain your solution more clearly.
   e) Written explanations (blurbs, in English) explaining important steps in your solution.
   f) The final answer, with units, sig-figs, and a box around it.
   

   See below for an example.
   
   Remember that this is Extra Credit: you're trying to impress the instructor with how well you can do. If in doubt, do a little more than you think you should, rather than trying to get by with less, at the risk of losing points on your solution.
8. The instructor will be available to answer questions about the assignment on a limited basis: before school, after school, and possibly by e-mail. The instructor will not be able to help you if you leave all of your work until the night before the assignment is due.

EXAMPLE PROBLEM AND SOLUTION:

SAMPLE PROBLEM:

In "The Matrix," Neo is given a test by Morpheus, who asks him to leap from the top of one building to another. Assume the buildings are each 100m tall, and 15m apart. Neo runs and leaps from the first building with a velocity of 5.00 m/s at an angle of 36.9 degrees (toward the second building).

a. Will he make it to the next building?
b. Where exactly will Neo land?

(Assume no air friction in this problem.)

EXTRA CREDIT PROBLEMS

1. Drag Race

A vehicle moving in a straight line can have its motion described as a function of its acceleration, but it is also the case that moving vehicles have a "top end," a maximum velocity that they can't exceed once they've reached it.

The drivers for Car 1 and Car 2 agree to have a "drag race" along a 394.9 meter length of straight road, accelerating from rest at one end of the road. Car 1 has a constant acceleration of 17.6 m/s² until it reaches its top end of 30.0 m/s, while Car 2 has a constant acceleration of 19.0 m/s² until it reaches its top end of 24.3 m/s.

1. Perform calculations that will identify which car makes it first to the end of the road.
2. Identify how much time it took the winner to arrive at the end of the road.
3. Determine how far apart the two cars were when the winner reached the end of the road.

2. Car on a Banked Turn

A car of mass 985.9 kg is traveling around a horizontal circle with radius 82.1 m, banked toward the center of the curve at an angle of 28.4 degrees relative to the horizontal.

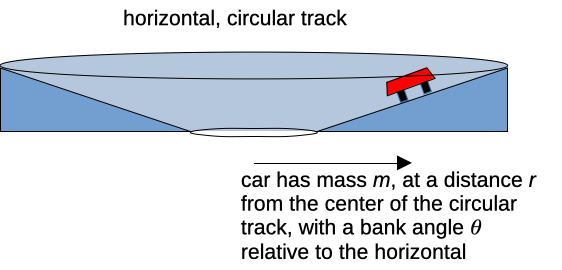
1. Assuming no friction is necessary to keep the car on the road, calculate the velocity of the car.
2. The coefficient of static friction between the car's tires and the road is 0.7 . Calculate the maximum velocity the car can have while still maintaining its circular motion around the track.

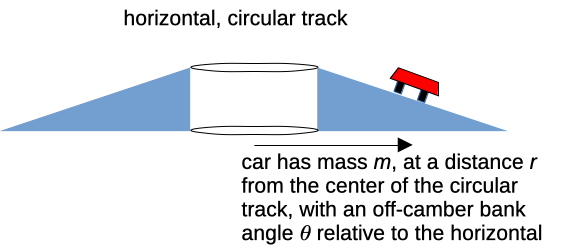
3. A car with the same mass, radius of motion, and coefficient of static friction travels along an off-camber track, where the track is tilted away from the center of the curve at 28.4 degrees. Calculate the maximum velocity this car can have while still maintaining its circular motion.

3. Water as a Projectile

A stream of water from a water fountain can be considered a series of water droplets moving together, each droplet tracing out the parabola of projectile motion. For this problem you'll be having a photo taken of you near a water fountain.

1. Before taking the picture, identify what "parallax error" is. Cite your source.
2. Have someone take a picture of you at the water fountain at Poly — be sure to include a meter stick and your face in the photo! What are you doing specifically to minimize parallax error in your picture?
3. By analyzing the photo/video and using the measuring device as a scale, you can make various length measurements (horizontal and vertical) to help you solve the other parts of this problem. Draw on the photo any lines or guides that indicate measurements you are using in your analysis.
4. Use your measurements (not a protractor) to calculate the x and y components of the water's velocity at the point where the water leaves the fountain.
5. Calculate the amount of time it takes for the water to reach its highest point.
6. Determine the angle (relative to the horizontal) of the initial velocity at the point where the water leaves the fountain nozzle by two different methods, and calculate the percent difference between the two values.

   Method 1: Measure the angle on the photograph using a protractor.
   
   Method 2: Calculate the angle based on your answers to part (d) above.

7. Develop a single equation to describe the water's trajectory (x and y coordinates) as a function of time, and express it using i, j notation.

4. Friction on a Jet Sled

You've built a jet sled that rides on rails, and that allows a rider to accelerate horizontally. The force from the jet engine is equal to 6.9 times the weight of the sled and its rider, mg, where the mass of the sled and rider are 237.6 kg.

There is also a force of friction between the sled and the rails which increases linearly as a function of the sled's velocity.

1. Draw a free-body diagram of the sled as it is being ridden.
2. Derive an expression for the sled's maximum speed, v—this expression should include an undefined constant of proportionality, k.
3. Derive an expression for the sled's velocity as a function of time, assuming it starts from rest.
4. Determine the velocity of the sled at:
   1. t = 0
   2. t = infinity
5. Derive an expression for the sled's position as a function of time, assuming it starts at the origin.
6. You are given a graph of the velocity as a function of time, but the graph is not complete. Assuming the mass of the sled and its rider is 237.6 kg, use the information provided to determine the value of k.